

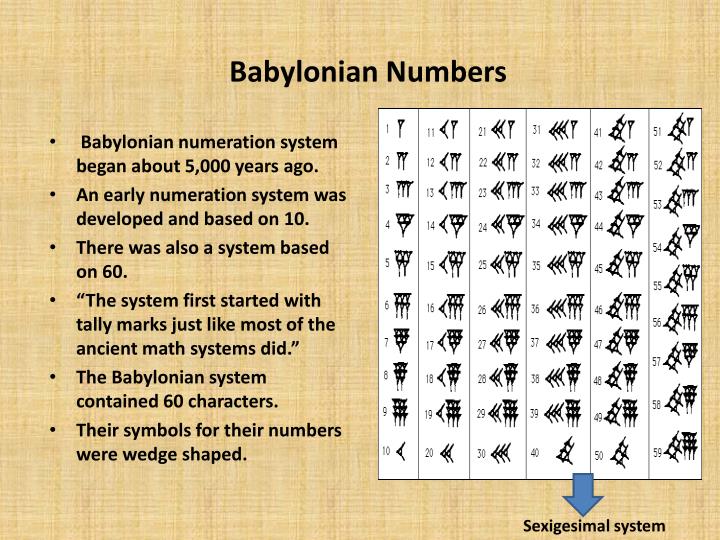
As we’ve already seen, the value of “2” depends on its position. Place-value notations, on the other hand, do require a placeholder digit. People might need a symbol to express “nothingness”, but the number system itself doesn’t require a numeral zero to function. Additive systems of numeration don’t require the use of a sign to denote zero. One remarkable thing you might have noticed in all these systems is that there is no numeral for zero. This is a critical element in the development of zero and we will get back to it. By 2000 BC, the Babylonians were using a powerful Base-60 positional notation with different symbols to represent specific quantities 9 Ifrah 2000:146. Starting in the 4th millenium BC, the Sumerians developed more sophisticated number systems along with cuneiform writing 8 Ifrah 2000:77-133. Faced with these issues many early civilisations did experiment with various grouping solutions 7 Ifrah 2000:7-8, but bureaucratic needs required more efficient number systems. It’s all well and good with small numbers but when the quantities you are recording become larger, you end up with an awful lot of bars (imagine having to write “1000” only with bars!). And to make it even easier, you can make groups of five bars, four vertical and one across, to easily spot and tally groupings of five. One of the most intuitive solutions is to make use of pebbles (or dots when writing) or twigs (bars): one vertical bar to represent one, four bars for four, ten bar bars for ten, etc. And it had to be invented.Īs a matter of fact, for the vast majority of our history, humans didn’t understand or use the number zero.įor basic mathematical tasks such as counting cattle, you would mainly need an easy and convenient way to record numbers and operate additions and subtractions. Natural numbers such as one, two, or three have a sensory equivalent: 1 cow, 2 pigs, 3 hens, etc.
The ancient Maya who, of course, wanted to avoid this kind of regrettable confusion, came up with a clever numeral system of their own as we are going to see now. But what a huge deception when you realise that your salary is actually “1×400 + 9×20 + 15×1” (i.e. You happily sign the contract thinking you are going to get “19×400 + 1×20 + 5×1” (i.e 7225 in decimal notation). Imagine a Maya employer offering you USD1915. 0-9) to write numbers in a Base-20 system doesn’t make calculus any easier and can even create unfortunate situations. This is what happens with “seven hundred and fifteen” because, in a vigesimal system, 715 is “1×400 + 15×20 + 15×1”.Īdmittedly, using Arabic digits (i.e. In other words, we would need to reach “400” (20×20) before adding a unit to the next position (i.e. In a vigesimal system, however, “three hundred” is “15×20 + 0x1” because numbers progress in powers of twenty. The number “three hundred” is written “300” in a decimal system and it means “3×100 + 0x10 + 0x1”. That’s why it is called a Base-10 (or “decimal”) numeral system. In other words, the value of the position increases from right to left in powers of ten: 1, 10, 100, 1000, 10000, etc. After “9”, we need to move across to the next position on the left where one unit is added. In that system, ten digits (0-9) are used to write numerals.
That’s why it is called a place-value (or “positional”) notation. The value of the numeral is linked to its position. Take the number “200”, for example: “2” actually represents “two counts of one hundred” because the digit “2” is located in the “hundreds place”. In the Hindu-Arabic numeral system, the value of a digit (i.e. The most commonly used number system is the Hindu-Arabic notation which reached Europe in the 11th century AD. To explain what a vigesimal system is, it is useful to start from something more familiar.


 0 kommentar(er)
0 kommentar(er)
